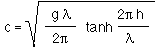
(valid for Δ < < 1, or λ > > H)
Waves are periodic deformations of an interface. Surface waves in oceanography are deformations of the sea surface, ie the atmosphere-ocean interface. The deformations propagate with the wave speed, while the particles describe orbital or oscillatory motions at particle speed and remain at the same position on average.
In deep water, particle paths are circles. In shallow water, the particle paths flatten to ellipses (Figure 9.1). The change from deep to shallow water waves is observed when the wavelength λ becomes larger than twice the water depth h. A change in wave properties occurs also at λ = 20h. It is therefore useful to distinguish between
|_______________________________|________________________________|________________________
0 < λ < 2h < λ < 20h < λ
deep water waves transitional waves shallow water waves
or short waves or long waves
It is most important to note that the distinction between deep and shallow water waves has little to do with absolute water depth but is determined by the ratio of water depth to wave length. The deep ocean can be shallow with respect to waves provided the wave length exceeds twice the ocean depth. This is the case for example with tides.
Ocean waves can be classified in various ways. One classification uses the forces which generate the waves. In ascending order of wave lengths we have:
The simplest way of looking at waves is the concept of a wave as a harmonic oscillation (Figure 9.3). It can then be described by its
In normal dispersion, c increases with λ, ie the crests of long waves travel faster than the crests of short waves. As a consequence cg < c, ie energy travels slower than the wave crests. This occurs with gravity waves such as swell.
Here, cg = c, ie all wave crests travel at the same speed, and energy propagates at the same speed.
This is found when cg > c. Capillary waves are an example. Energy propagates faster than wave crests, and short waves travel faster than long waves.
In most oceanic situations the wave steepness is very small and the wave speed is then given by
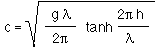
(valid for Δ < < 1, or
λ > > H)
Here, H is the wave height and h the water depth. The formula can be simplified depending on the ratio of λ against h:
| Deep water wave speed (short waves; depth is larger than 1/2 of the wavelength) |  | cg = c / 2 (normal dispersion) |
| Shallow water wave speed (long waves; depth is less than 1/20 of the wavelength) | 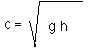 | cg = c (nondispersive waves) |
As an example, consider waves produced by a distant storm. In the open ocean such waves travel as deep water waves; their wave speed therefore depends on their wavelength λ. Thus long waves travel fastest and arrive at distant locations first. They are experienced as swell. When swell approaches a beach the water depth decreases and there comes a point when the waves change from deep water waves to shallow water waves. As a consequence the wave speed c decreases as the depth decreases and the waves bend inwards; the wave front becomes progressively more parallel to the beach.
Waves of finite amplitude
The harmonic oscillation concept gives a good description of waves of very small steepness. On the approach of a beach, or during the period of active formation by wind, wave steepness is not small enough and the wave profile deviates from the harmonic profile. As the steepness increases, the wave profile becomes cusped:
Eventually, the waves break. The limiting values before breaking are 120° for the cusp angle and a steepness Δ = 1/8. Cusped waves do not have closed particle paths but are associated with a net transport of water (not just energy). This transport is called Stokes drift, after its investigator.
Short waves (deep water waves)
Short waves in the ocean are wind generated waves. They are divided into sea and swell. Sea includes all waves generated by the local winds, while swell refers to waves generated by distant wind fields.
The effect of the wind on the state of the sea depends on the distance over which the wind can blow without hindrance before it reaches the observation point. This distance is known as the fetch. The presence of a coast limits the fetch for winds blowing from land to sea. In the open ocean the fetch is usually determined by the size of the weather system that produces the wind.
Another wave determining factor is the time during which the wind blows unabated with a given strength. At any given wind speed it takes a certain time for the waves to build up to a steady state. The time required to reach that state (where the waves do not grow any further) is known as the duration of the wind.
At any given instant in time the sea state is never a single harmonic oscillation, but appears to the observer as a chaotic process. Therefore ways have to be found to describe the wave conditions in terms of measurable statistical quantities. Two approaches are used:
1) Determination of significant wave parameters (description in the time domain)
2) Determination of the wave spectrum (description in the frequency domain)
The combination of many measurements has resulted in wave parameter estimates for fully developed seas (Figure 9.5). A fully developed sea is one for which the fetch and duration are not limiting ie no further growth occurs, as momentum and energy loss through breaking balances input from the wind.
The graph shown in Figure 9.5 applies to situations where waves are generated by local wind. Figure 9.6 shows wave properties for situations where the waves are generated remotely. It assumes fully developed sea conditions in the generation region.
For many marine applications, for example the routing of ships or the design of platforms, only the heighest waves are of interest. The quantity significant wave height has therefore been introduced. It is defined as either H1/3 or H1/10, ie as the average of the 1/3 or 1/10 heighest waves over an observation period. (The use of H1/3 is more common than the use of H1/10). From observations, the largest wave height Hmax is related to the significant wave height by
Measured maximum wave heights depend to some degree on the length of the observation period; different values for Hmax are found from a 10 minute time series compared to a 3 hour time series.
The relations between largest wave height and significant wave height indicate that the sea state has certain statistical properties. A statistical description is based on the representation of the wave field through the energy spectrum. For a given frequency, wave energy is proportional to the square of the amplitude.
An energy spectrum shows wave energy as a function of wave frequency. A single harmonic wave has a 'monochromatic' spectrum:
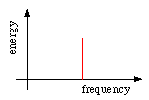
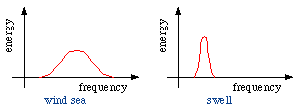
Statistical description assumes that waves of all frequencies and corresponding wavelengths are present. It does not attempt to describe the form of the sea surface but concentrates on wave energy. In a wind sea with random distribution of wave energy over all wave frequencies, the theoretical form of the energy spectrum is that of a Gaussian or 'normal' distribution. Where only swell is present the energy is concentrated near the swell frequency and the spectrum is much narrower:
In real oceanic situations energy is not randomly distributed but cascades from the shortest possible waves stirred up by the wind to the longer wavelengths. As a result the shape of ocean wave spectra depends strongly on the wind speed. Figure 9.7 shows observed energy spectra for fully developed seas at various wind speeds. Note that the spectrum has a normal distribution only for very low wind speeds; as the wind speed increases, waves of short period are still present but most of the energy is found in longer period waves. The spectrum then drops off rapidly at longer periods; it is "skewed" towards the longer periods.
Final wave decay occurs during breaking on a beach, which occurs when the particle velocity becomes larger than the phase velocity (wave speed). In this state the waves transport both energy and mass towards the beach. While much of the energy is dissipated in mechanical reworking of the beach, the mass moved by the waves has to be returned to the sea. This occurs at regular intervals along the beach in so-called rip currents, strong seaward movement of the water along the sea floor ("undertow").
contact address: